I ran this back on 2010 as part of my W&T series. I’m including it here for completeness.
God came from Teman… He stood, and measured the earth. (Habakkuk 3:4,6)
Who hath measured the waters in the hollow of his hand, and meted out heaven with the span, and comprehended the dust of the earth in a measure, and weighed the mountains in scales, and the hills in a balance? (Isaiah 40:12)
In my first post over at Wheat and Tares (check it out if you missed it), I mentioned one of my favorite books, Mathematics for the Million. I also took an example from it on how to calculate the value of PI using reason.
This book also gives several interesting examples of other things that you can do with math. Did you know that you can mathematically calculate the size of the earth, the distance to the moon, and the size of the moon? Did you know that if you know the trick, you can form a right angle without a tool?
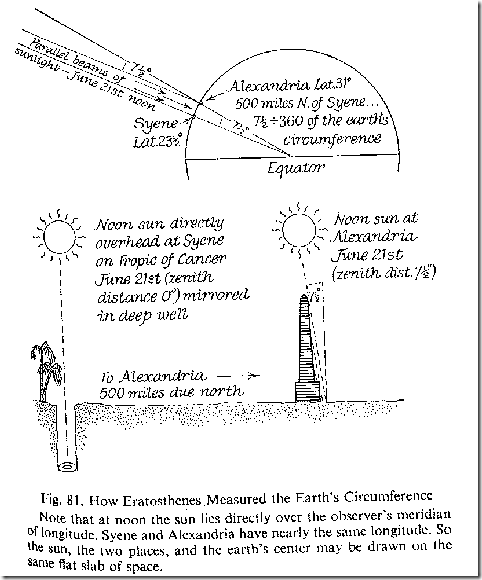
Measuring the Earth’s Radius and Circumference
The trick to measuring the Earth’s circumference is to find a well that the sun directly passes over so that you can see the reflection at the bottom of the well. This can only happen on the tropic of cancer, and only on June 21. At the same time that happens, also measure the angle of the shadow at some distance away but at the same longitude. The book uses the example of Syene and Alexandria.
Now all you have to do is use Trig to figure out the angles and sizes of the triangle that forms between the earth’s center and the two cities. It’s a bit of work, but it’s obvious that it will work. [1]
Measuring the Moon
It’s even easier to measure the moon. Now that we know the radius of the earth, we can take measurements on a single longitude of the angle of the moon. Now we use Trigonometry to calculate the distance to the moon.
By measuring the angle of the top and bottom of the moon, we can now measure the size of the moon itself.
Ancient Right Angles
This one is my favorite. Suppose you wanted to measure out a right angle, but didn’t have a compass or square because they hadn’t been invented yet. Could you do it using only high school mathematics? You can, actually, you probably just haven’t thought it through all the way.
Do you remember learning in high school that all right triangles (i.e. a triangle with one of its angle at a right angle) will follow the Pythagorean theorem?
(A^2)+(B^2)=(C^2)
One of the easiest solutions for the Pythagorean theorem (and one of the most common right triangle ratios on the GRE) is the 3:4:5 ratio. Try it:
(3^2) + (4^2) = (5^2)
9+15 = 25
So if you need a right angle, just take any measure you want and mark out on a rope 3 units, four units, and five units. Now stake the marked points down. Viola! Instant right angle. No wonder Pythagoras founded a secret cult and tried to hide his mathematical knowledge from the world.
Again, we see how mathematics is just simple logical steps that build up into something complex and beautiful. Yet when we are done, we know its right. There just isn’t any question.
It’s also interesting that these are all areas that, as the Bible quotes above indicate, were once the sole domain of God.
Notes
Images are from Mathematics for the Million, pages 207, 228, 229 respectively.
[1] See Mathematics for the Million p. 207 for details.