I believe people willing to persevere through this post will find themselves surprised by the end by the rather starling philosophical implications of quantum physics. I also believe that, if you take it slowly, the math is understandable to any high school graduate. I am personally very bad at math and can only handle this example because the math is so easy. If you don’t assume you can’t understand it, you’ll find that you can.
Forget What You Think You Know
Unless you are a physicist, start by emptying your mind of what you think you know about quantum physics through popular books because there is a substantial gap between what people say about Quantum Physics and the real theory. It seems to me that Quantum Physics currently gets used as the new ‘magic’. It’s become common for the fad magical (or sometimes even religious) worldview of the moment to slap a ‘quantum’ label in there somewhere to add a scientific veneer. [1] The reason this happens is because quantum physics has a deserved reputation for being really ‘weird’. But keep in mind what ‘weird’ means. It only means “something I’m not familiar with.” Claiming something is ‘weird’ says nothing ontological about the object/idea in question and actually serves as a statement about the speaker’s state of ignorance of the subject. (A point I often bring up when we talk about Mormons or other religions being “weird.”)
Contrary to popular belief, it is not entirely true that quantum physics allows for an object to be in two places at once. This is only true in a very limited sense which I’ll explain as part of this post. It is also not true that quantum physics necessarily enshrines human consciousness as the center of reality. Amit Goswami’s popular science books aside (i.e. The Self-Aware Universe), once you understand how Quantum Physics really works I suspect you’ll agree that the case for the centrality of consciousness in Quantum Physics is lacking.
The Dud Bomb Problem
I’m going to use the “Elitzur-Vaidman Bomb Tester Problem” to teach the basics of quantum physics. I also choose it because it’s implications are startling.
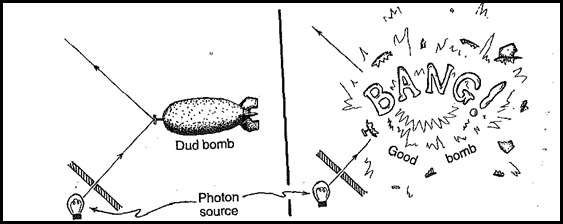
Pretend that there is a group of bombs that are triggered by a single photon bouncing off a small mirror at the nose of the bomb. If the bomb is not a dud, the bomb explodes when the photon hits its trigger mirror. See illustration below.
So the problem to be solved is this: come up with a way to prove that a bomb is not a dud without causing it to explode?
This reminds me of the old Bugs Bunny cartoon where Bugs is on an assembly line and is hitting each bomb and, if it doesn’t explode, he writes “dud” on it. In classical physics, this problem is as unsolvable as it was for Bugs. But we live in a quantum world not a classical one, so classic physics isn’t reality. In reality, it is possible to test these bombs in such a way so that we can collect together a group of bombs that we know are not duds yet haven’t, as yet, been exploded.
How? It’s impossible, right? That’s what makes quantum physics so dang strange. It’s utterly at odds with our intuitions about reality.
By the way, according to Wikipedia, this experiment (or a version of it) has been tested in reality.
Part 1: Quantum Phenomenon and Half Silvered Mirrors
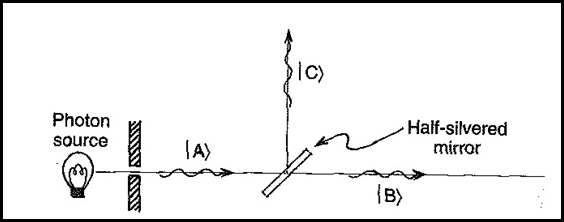
Now start by looking at the illustration below.
In this illustration we have a photon source that emits a single photon that shoots out and hits a half-silvered mirror. A photon is a single ‘particle’ of light. It’s the smallest amount of light that can be emitted. It turns out that light is ‘lumpy’ because there are minimum quantities that can be emitted. That is to say, light is discrete, not continuous. It’s like pixels on a computer screen: it comes in a minimum size.
A half-silvered mirror is a mirror that has just enough ‘silvering’ on it such that there is a 50/50 chance that the photon will bound off of it vs. pass through it.
Now pretend like we add a photon detector at points |C> and |B>. What would you intuitively expect to happen? You’d expect to either detect a photon at point |C> or at point |B> right?
In this case, your intuition is correct. But I want you to mathematically understand why this is true using the quantum formalism.
Mathematically we’d write it like this:
|A>
Or, at least that is the closest I can get to the right symbols on a web page. Even using Microsoft’s “Math Input Panel” wasn’t enough to get it perfectly right.
But what does that funny looking equation mean?
Explaining the Math
The first thing to know is that the symbol “
Because it’s so difficult to write this all out for a web page, I’m going to simplify it further. For the example I’m using, I don’t really need the “|” and “>” so I’m going to drop it from here on in. So our formula now reads:
A
Now that you know that “
Imaginary Numbers
Here, you’ll need to harken back to your junior high school days when they taught you about something called “imaginary numbers” and you scratched your head and wondered why they were teaching you this when it was clearly useless and you’d never use it again. Or at least that is what I remember thinking about imaginary numbers.
What is an “imaginary number”? It’s essentially a number that contains a negative square root.
Yup, you heard me right. “Imaginary” numbers consist of numbers like
So the brainy mathematicians realized that to work with imaginary numbers you really only need to add a symbol for “
So what, exactly, is
But as it turns out, it doesn’t actually matter if you can envision -1 in your mind or not. All you really need to know is how to do mathematical functions with it like add, subtract, multiply, and divide with it. The same is true for
Back At Our Formula
So getting back to our formula now, the photon at state A has now evolved into state B + iC when it hit the half silvered mirror. This B + iC is typical of how brainy mathematicians write down imaginary numbers. They split them up into a normal number part (in this case “B”) and an imaginary part (in this case “C”). The end result is B + iC.
Frankly, I’m a bit unclear why we let “B” be the normal part of the formula and “iC” the imaginary part of the formula. This is really just something that is inherent to the nature of quantum physics. Apparently the path of the photon reflecting ends up being the “imaginary” part of the formula. Really, this won’t matter so much as I’m copying this out of a book from a brainy physicist, so we can trust he’s got it right and move on.
Probability Waves
But what does that formula even mean? Well, that’s the other thing you need to know to understand quantum physics. Quantum physics is actually built up out of probabilities. When the photon hits the half silvered mirror we said there was a 50/50 chance as to which point it would go to. What did we mean by this? It’s not quite what you’d imagine.
What we actually do is we then take the state of the formula (i.e. in this case B + iC) that we’ve ‘evolved’ to and when we take a measurement (i.e. see if the photon reached point B or C) we take the ratio of the two states to determine the ‘probability’ of the chances we’ll detect the photon at either point B or C.
So, for our current state of B + iC the ratio is, of course, 1:1. So there is a 50% chance we’ll detect the photon at point B and 50% at point C – exactly as our intuitions would lead us to believe.
Wow, what was an awful lot of work for what ultimately was an intuitive answer, wasn’t it? But hold on to the seat of your pants because your intuition is about to go out the window.
Part 2: Add Another Half Silvered Mirror and 2 Full Silvered Mirrors
Okay, let’s now imagine that we’ve decided to add to the experiment so that it now looks like this:
Basically we’re going to reflect the photon off one of the two full silvered mirrors (i.e. it’s got 100% chance of bouncing and 0% chance of passing through) and finally both paths will converge on a final half-silvered mirror (i.e. 50/50 chance of bouncing or passing through.)
What does your intuition tell you is going to happen now?
Mine tells me that there is now a 50/50 chance that the photon will be at either point G or F, right? If you agreed, go to the back of the room and put on the dunce cap! Because your wrong! To see why this isn’t the case, we have to do that quantum math.
We were previously in state: B + iC. Now, based our experiment, we should expect that B
B + iC
Oh, that’s a bit harder to look at, isn’t it? What the heck does i(iE) mean?
But it looks scarier than it is. Remember that multiplication is transitive which means you can do it in any order. So i(iE) just means
iD – E
What’s next? Well, it’s exactly what you’d guess. E evolves to be F + iG and D evolves to be G + iF. So let’s plug that into the formula and see what we get:
iD – E
Now simplify it down, first by spreading the first “i” over the (G + iF). That would be the same as iG + iiF which we know is the same as iG – 1F or in other words:
iG – F
Now add in the other part of the equation:
iG – F – (F + iG)
Finally, simplify:
= iG – F –F – iG
= iG – iG – F – F
= -F – F
= -2F
So our final state is “-2F”.
Wait! The G is gone!
It just so happens that the way this experiment is setup, the G ceases to exist due to the way imaginary number math works. Because there is no G, the ‘ratio’ between G and F is essentially 0:1. Or in other words, there is now – according to quantum theory – a 100% chance that the photon will show up at point F and 0% chance it will show up at point G! And this is precisely what happens in real life via experiment.
Quantum Weirdness
Now that is counter intuitive isn’t it? Why in the world does setting up an experiment with two paths like this happen to cause the photon to always come out at point F and never at point G? This counter intuitive phenomenon is what is known as “quantum interference.” The idea is that both paths for the photon actually, somehow, interfere with each other.
Okay, okay, let’s head off any objections. It’s just a theory right? Yeah! One that has been replicated countless times. If you actually try this in a laboratory, this is the result you get! The photon always comes out at point F!
Let’s Add a Rock
Now suppose you decide to change the experiment around by placing a rock in place of the full silvered mirror between B and D. Like this:
What happens now? You can work the math out if you wish. The bottom line is that there is now a 50% chance the photon will hit the rock (as per part 1 above) and a 50% chance that it will hit the full-silvered mirror between points C and E. If it does hit the full-silvered mirror between C and E, then, of course, there is a 50/50 chance it will come out at either point G or point F. So the odds are these then:
50%: hits the rock and we don’t detect anything
25%: hits point G
25%: hits point F
Stop and let that sink in for a moment. Why is it that having the rock not blocking one of the paths changes things to cause it to always hit point F! Why doesn’t it do the intuitive thing and just work out to be 50/50 of being G vs. F?
The answer to that question is stunning: somehow the photon knows whether or not the path through B and D is open or not!
In Two Places At Once?
This is the limited sense in which quantum physics says that the photon is in two places at once.
Quantum physics never allows for you to actually see the photon in two places. The real truth is that it just somehow knows that the other path (the one it didn’t take) was open to it. It is influenced by that other path that it didn’t take. I can see why some scientists refer to this as the photon being in two places at once, but I’m uncomfortable with this interpretation because I feel it intuitively leaves a false impression about what really happens. To me, if the photon is “in two places at once” then the wave function collapse – as I’ll explain in the next section – shouldn’t happen. Yet it does.
Wave Function Collapse: Quantum Physics and “Observation”
Now let’s change the experiment just a bit. Let’s put back the mirror between points B and D, but let’s add a little detector to the mirror so that we can see if the photon hit that mirror or not before bouncing along the path. So it now looks like this:
This setup is identical to the one above (before we added the rock) isn’t it? The photon again has both paths open to it. So what would be the outcome?
If you’re like me, you probably guessed that again we end up with the photon only exiting at F, just like before. In other words, you’re predicting that the interference of the second path starts to happen again because the path is open again. But this isn’t what happens!
The mere act of adding the detector cause the interference pattern to disappear – just like if there was a rock there! So the photon starts to exist at G half the time and at F half the time, just like our original intuitions thought it should.
To understand why this happens, you have to know one more thing about quantum physics: the act of observing causes the whole quantum evolution process to start over again. What this means mathematically is that once the photon hits the mirror between B and D (and is detected) the evolution of the path through C and E ceases to exist! This phenomenon is known as the ‘wave function collapse.”
Strangely, if the photon does take the C and E path the very fact that the B and D path would have detected it causes that path to cease to exist also.
As before, our evolution starts out looking like this:
A
But once the photon reaches either of the full-silvered mirrors we, in theory, can know which mirror the photon bounced off of. If the detector between B and D registers a photon, then we know that is the path the photon took. But if the detector does not register the photon, then we can be sure that the photon took the C and E path. Either way, we know (after reviewing the detector data) for sure which path the photon took. The very fact that we know have this data available causes the evolution of the equations to start over.
What this means mathematically is that if the photon hit the mirror between B and D, that becomes the start of a new evolution process. Likewise for if the photon bounced between the C and E mirror. So we’ll either end up with B
Because the evolution of the quantum equations starts over, the interference pattern also disappears now. To demonstrate why this is, do the math. Where we left things was that we either had B
You can work out the math from here now:
Either:
iD
For both of these possible evolutions, the ratio between F and G is again 1:1. So we’re back to having a 50/50 chance of getting either F or G. The interference pattern is gone!
Notice something else here: the photon detector was the ‘observer’ here, not a human being. “Observation” in quantum physics is never specifically a conscious being observing something, as Amit Goswami (and others) might have you believe. Quantum physics does not appear to put human consciousness at the center of reality. Any sort of detector (or as we saw, even a rock) will do.
This is also why I’m hesitant to say that in quantum physics a photon or particle can be in two places at once. The fact is that the photon only takes one of the two paths. It is (as we’re about to see) really just the fact that the photon could have taken the other path but we don’t know which path it took that causes the existence of an interference pattern. Once you throw a detector on there, there is never ever a case where you can detect the photon taking both paths at once.
Testing For a Dud
I have now given you all that you need to solve the test bomb problem. Simply take the bomb, with its little mirror on the front, and make it the full silvered mirror between B and D. Like this:
Now, according to quantum theory, what happens? Feel free to do the math for if the bomb is a dud vs if the bomb is not a dud. I would appear something amazing happens.
In the case where the bomb is a dud, our experiment is exactly like not having a detector, for the bomb does not explode when the photon hits its mirror because it’s a dud. But if the bomb is not a dud, the bomb functions as a detector and the interference pattern vanishes!
So here is the key now: what does it mean if you shot a photon at the above experiment and you get a detection at point G?
It means you know for sure the bomb is not a dud even though the bomb did not explode!
The Truth about Reality: Counterfactuals vs. Many Worlds
Roger Penrose suggests that the best way to think of Quantum Physics is as somehow dealing with counterfactuals. A counterfactual is actually a familiar concept. It is not uncommon to say something like “had Romney not been a Mormon, John McCain would not have received the Republican nomination in the 2008 election.” What does a statement like this really mean? From a classical physics point of view, it means very little. Since Romney is a Mormon the statement is (logically speaking) factually false at the outset. [2] Its meaningless. Or is it?
Intuitively, we ‘get it’ that a counterfactual statement is not meaningless, though trying to explain what we really mean by it is a bit difficult to explain. We just mean that, had there been some other reality the same as ours save only that Romney was not a Mormon, the outcome of the 2008 primary election would have been different. (Did that explain it any better? Not really.)
What quantum physics is telling us, according to Roger Penrose, is that if the photon comes out at G, we know for certain that counterfactually, had the photon taken the B to D path, the bomb would have exploded. Thus we know it is not a dud. In short, counterfactuals somehow ‘physically exist’ in reality, at least in a case like this.
David Deutsch, on the other hand, would say that we live in a multiverse of many realities and that what actually happened is that in 50% of the alternate realities out there, the bomb did explode. That reality then decohered from ours and could no longer communicate with us and that is why we got the photon at point G. (i.e. that is why the exploding bomb acted more like the rock. It caused that reality and ours to stop communicating.)
It’s not hard to see that no matter which of these is the truth, there are significant philosophical ramifications.
The simple truth is that: quantum physics says something about reality that is wholly counter intuitive and simply fantastical. It is either saying something about counterfactuals being (in some sense) physically real or its saying we live in a multiverse of realities. Either way, if you are like me, your mind is probably blown out of your head and lying on the floor behind you. Excuse me while I pick mine up again and shove it back into my head.
P.S. Doing a post like this is quite difficult. So anyone that reads this that understood it even partially and enjoyed it and wants to see more such posts (and I’m assuming we’re not talking about a very large crowd) please be sure to put a comment if only “read and enjoyed by no comment”. This will give me an indication that there are a few people out there that really like this stuff as much as I do I’ll do more in the future. If you are a regular lurker, this might be a good time to make a comment for the first time in your life.
Notes
[1] The Secret is the prime abuse of quantum physics here. But also Barbara Hagerty, author of Fingerprints of God: What Science is Learning About the Brain and Spiritual Experiences wanted to use quantum entanglement to explain how a mother knows her child is in danger, etc. She suggests the entire universe is quantum entangled, etc. It makes one wonder why observing your child every day since birth didn’t collapse the wave function and end the entanglement.
[2] Well, actually, that’s not really true. The real truth is that if you make a statement “if X is true, then Y is true” and X is not true, then the whole statement is actually considered logically true. So that means the statement is factually true, not factually false. Just not in the way I meant it in that context.